多复变与复几何

9. 湖南大学李木林教授12月5日(周日)19:00-20:30

题目和摘要如下：

Title: Deformation rigidity of minimal projective manifolds

Abstract: Let $\pi:X\to \Delta$ be a smooth family of compact complex manifolds. 

Suppose that $X$ is K\"ahler and the fibers $X_t$ are biholomorphic to $S$ for all $t\in\Delta^*:=\Delta\setminus 0$, 

where $S$ is a fixed complex manifold. We prove that the central fiber $X_0$ is biholomorphic to $S$, 

when $S$ satisfies some additional conditions.

报告链接

https://meeting.tencent.com/dm/KLPCueaw6mEQ

8.  Institute for Basic Science, Center for Complex Geometry 李起峰博士11月29日(周一)19:00-21:00

Title: The VMRT structure and its applications

Abstract: By the VMRT, we mean the varieties of minimal rational tangents on a uniruled projective manifold. It is an invariant of a uniruled projective manifold. Conversely, the VMRT structure contains rich information of the geometry of the manifold. In this talk, I will discuss on the VMRT structure and the application to the geometric problem, such as the deformation rigidity, of some concrete Fano manifolds. 

报告链接

https://meeting.tencent.com/dm/ivw5mIWYtcKe

会议ID：232 920 517

7.  Katholieke Universiteit Leuven 郝峰博士11月21日(周日)19:00-21:00

题目和摘要如下:

Title: holomorphic 1-forms and geometry of algebraic varieties

Abstract: In principle, holomorphic 1-forms of irregular smooth projective variety $X$ encode much information of the topology and birational geometry of $X$. 

In this talk, I will first give a survey on some works and problems about how the zeros of holomorphic 1-forms affect the birational property and topology of smooth projective varieties, and try to briefly recall some concepts on birational geometry and cohomology jump loci theory that are used in the later discussion. 

Then I will discuss a recent work (joint with Yajnaseni Dutta and Yongqiang Liu) on how the existence of nowhere vanishing holomorphic 1-forms on a smooth complex projective variety $X$ affect the singularities of morphisms from $X$ to (simple) abelian varieties. 

For the last part, I will try to discuss an in-progress work on the classification of smooth projective varieties of Kodaira codimension one which admit nowhere vanishing holomorphic 1-forms.  

报告链接

https://meeting.tencent.com/dm/TIhzxlV3HODn

6.  重庆理工大学数学科学研究中心夏炜博士11月14日(周日)19:00-21:00

 题目和摘要如下:

题目：Deformations of complex structures and cohomology classes

摘要：I will introduce some recent progress related to the deformation theory of compact complex manifolds.

In the first part, I will recall the basics of classical Kodaira-Spencer theory, in particular, the power series point of view.

In the second part, I will talk about some recent progress about deformation of cohomology classes  and some related problems.

报告链接

https://meeting.tencent.com/dm/QuSCN9a1Si77

4. 首都师范大学数学科学学院朱智贤教授10月21号(周四)下午14：30-16：35

她的报告题目和摘要如下：

Title: 

           Fujita's freeness conjecture

Abstract: 

           Let $X$ be a smooth projective variety of dimension $n$ and $A$ any ample line bundle. 

Fujita conjectured that the adjoint line bundle $\mathcal{O}(K_X + mA)$ is globally generated 

for any $m$ greater or equal to $\dim(X) + 1$.  One of the standard techniques in the study of 

Fujita's freeness conjecture is an induction method, called cutting down the minimal log canonical center. 

In this talk, I will explain how to apply this method to prove Fujita's freeness conjecture in dim 4 and 5.  

 报告链接

 https://meeting.tencent.com/dm/BehFFgRSdbfO

3. 南开大学陈省身数学所李琼玲教授10月14号(周四)下午14：30-16：35

她的报告题目和摘要如下：

Title: 

            Nilpotent Higgs bundles and the non-Abelian Hodge correspondence

Abstract: 

           In the first hour of the talk, we introduce the basic notions like Higgs bundles, flat connections, 

representations, harmonic maps, stability, and the moduli spaces. Then we give a brief introduction to the 

celebrated non-Abelian Hodge correspondence theory. 

           In the second hour of the talk, we study an algebraic inequality for nilpotent matrices and show some 

interesting geometric applications: 

(i) obtaining topological information for nilpotent polystable Higgs bundles over a compact Riemann surface; 

(ii) obtaining a sharp upper bound of the holomorphic sectional curvatures of the period domain and the Hodge 

metric on the Calabi-Yau moduli. 

           If time permits, we also show a generalization of this work to n-Fuchsian fibers in the moduli space of Higgs 

bundles. Part of this talk is joint work with Song Dai (Tianjin University).

 报告链接

 https://meeting.tencent.com/dm/XD9tRa2lZNj3

2. 中山大学数学学院(珠海)周晨博士9月30日(周四)16：00-17：00线上报告。

周晨博士去年毕业于美国纽约州立大学布法罗分校，师从Mohan Ramachandran教授，

本科毕业于武汉大学，硕士毕业于南开大学。

他的报告题目和摘要如下：

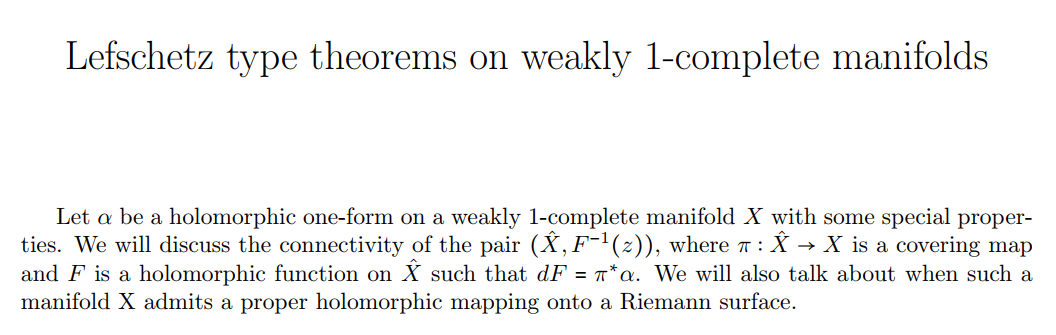
报告链接

https://meeting.tencent.com/dm/wztcl2FWKInx

 欢迎大家参加！

1. 北京大学袁铮博士9月23号(周四)下午 15：00-16：35线上报告，

主要内容为他和关启安教授、秘志桐博士最新的工作，

题目和摘要如下

报告链接

https://meeting.tencent.com/dm/RikZsf3PkvSK

5. (待定) 中科院数学研究所王隽永博士11月第一周

     Positivity of direct images and applications to birational geometry

10. 天津大学应用数学中心杨松教授12.11(周六)19：00-21：00

题目和摘要如下：

标题：Cohomology and bimeromorphic invariants of complex manifolds

摘要： This talk gives an exposition on cohomology (e.g., Bott--Chern, Dolbeault, twisted de Rham etc.) of compact complex manifolds 

and constructing bimeromorphic invariants of compact complex manifolds, and also on Hodge cohomology of smooth proper varieties 

over an algebraically closed field of positive characteristic. It is based on joint works with Youming Chen, Sheng Rao, Xiangdong Yang and Xun Yu.

报告链接：

腾讯会议#：234-158-327

https://meeting.tencent.com/dm/3AvSNgCDU9uf

https://space.bilibili.com/7237500/